Calculating integrals is a regular part of mathematics, particularly for calculating probabilities, which is fundamental to data science. Generally, it is necessary to know a primitive of a function in order to calculate its integral. In this article, you will discover the definition of primitives and how do you determine the primitive of a function.
What is the primitive of a function and how do you determine the primitive of a function?
Let \left[a,b\right] be an interval, and f : \left[a,b\right] \to \mathbb{R} a function defined on the interval \left[a,b\right]. We say that f admits a primitive on \left[a,b\right] if there exists a differentiable function F : \left[a,b\right]\to \mathbb{R} such that for any x \in \left]a,b\right[, F^{\prime}(x) = f(x).
We then say that F is a primitive of f.
For example, for f(x) = 3 x^2 + 5, a primitive of f on \mathbb{R} is F(x) = x^3 + 5 x. This can be verified by deriving F.
We then say that F is a primitive of f.
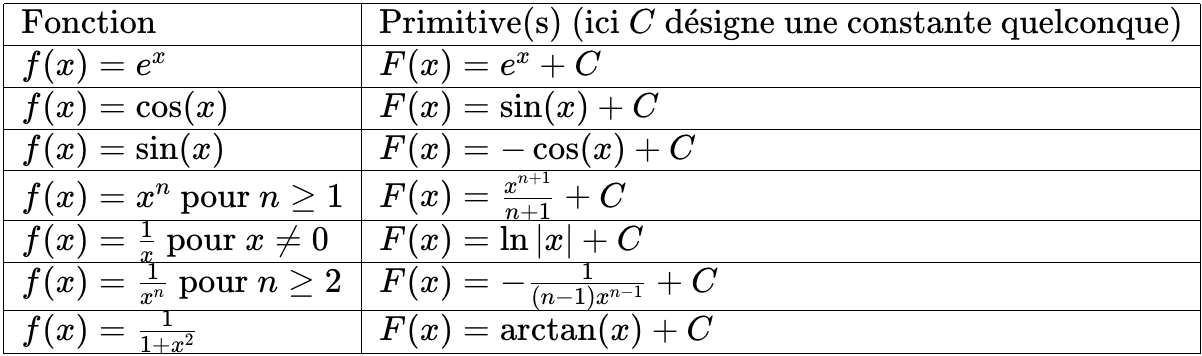
The following table shows the primitives of some common functions.
We then say that F is a primitive of f.
Cliquez sur le tableau pour l'afficher en plein écran.
Sufficient condition for the existence of a primitive
Let \left[a,b\right] be an interval, and f : \left[a,b\right] \to \mathbb{R} a function defined on the interval \left[a,b\right].
We then say that F is a primitive of f.
Let \left[a,b\right] be an interval, and f : \left[a,b\right] \to \mathbb{R} a function defined on the interval \left[a,b\right].
We then say that F is a primitive of f.
In this case, F is the only primitive of f that cancels at a. This result is known as the fundamental theorem of analysis.
So, if a function is continuous over an interval, it admits a primitive over the interval.
Integral and primitive relationship
Knowing a primitive of a function f allows you to calculate its integral over segments.
Indeed, if f is a continuous function defined on \left[a,b\right] and if F is a primitive of f, then we have
\int_{a}^{b} f(x)dx = {\left[F(x)\right]}_a^b = F(b) - F(a)
\int_{a}^{b} f(x)dx = {\left[F(x)\right]}_a^b = F(b) - F(a)
Properties on primitives
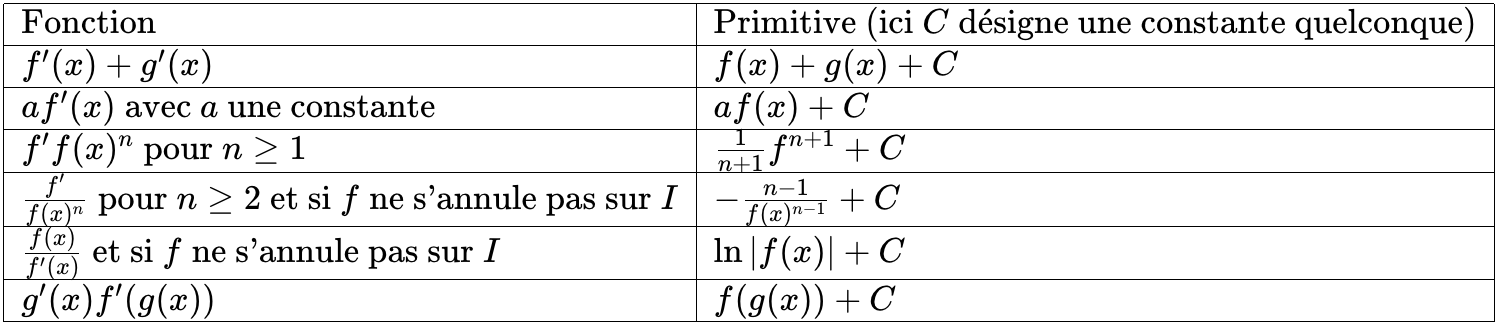
It is possible to state a number of relationships which follow from the derivation formulae. Here we consider two derivable functions f and g defined on an interval I. The table below summarises the primitives of the main operations on functions.
Cliquez sur le tableau pour l'afficher en plein écran.
You now know what a primitive is and how do you determine the primitive of a function. Primitives are mainly involved in the calculation of integrals, and are closely related to the derivation of functions.
If you would like to discover all the mathematical concepts involved in data science, we invite you to take a look at our courses.